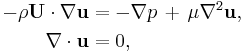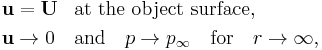Oseen equations
In fluid dynamics, the Oseen equations (or Oseen flow) describe the flow of a viscous and incompressible fluid at small Reynolds numbers, as formulated by Carl Wilhelm Oseen in 1910. Oseen flow is an improved description of these flows, as compared to Stokes flow, by the partial inclusion of convective acceleration.[1]
Using the Oseen equation, Horace Lamb was capable to derive improved expressions for the viscous flow around a sphere in 1911, improving on Stokes law towards somewhat higher Reynolds numbers.[1] As well as for the first time deriving a solution for the viscous flow around a circular cylinder.[1]
The Oseen equations are, in case of an object moving with a steady velocity U through the fluid — which is at rest far from the object — and in a frame of reference attached to the object:[1]
where
- u is the disturbance velocity induced by the moving object, i.e. the total flow velocity in the frame of reference moving with the object is –U+u,
- p is the pressure,
- ρ is the density of the fluid,
- μ is the dynamic viscosity,
- ∇ is the gradient operator, and
- ∇2 is the Laplace operator.
The boundary conditions for the Oseen flow around a rigid object are:
with r the distance from the object's center, and p∞ the undisturbed pressure far from the object.
Notes
References
- Oseen, Carl Wilhelm (1910), "Über die Stokes'sche formel, und über eine verwandte Aufgabe in der Hydrodynamik", Arkiv för matematik, astronomi och fysik vi (29)
- Batchelor, George (2000), An introduction to fluid dynamics, Cambridge Mathematical Library (second paperback ed.), Cambridge University Press, ISBN 978-0-521-66396-0, MR1744638